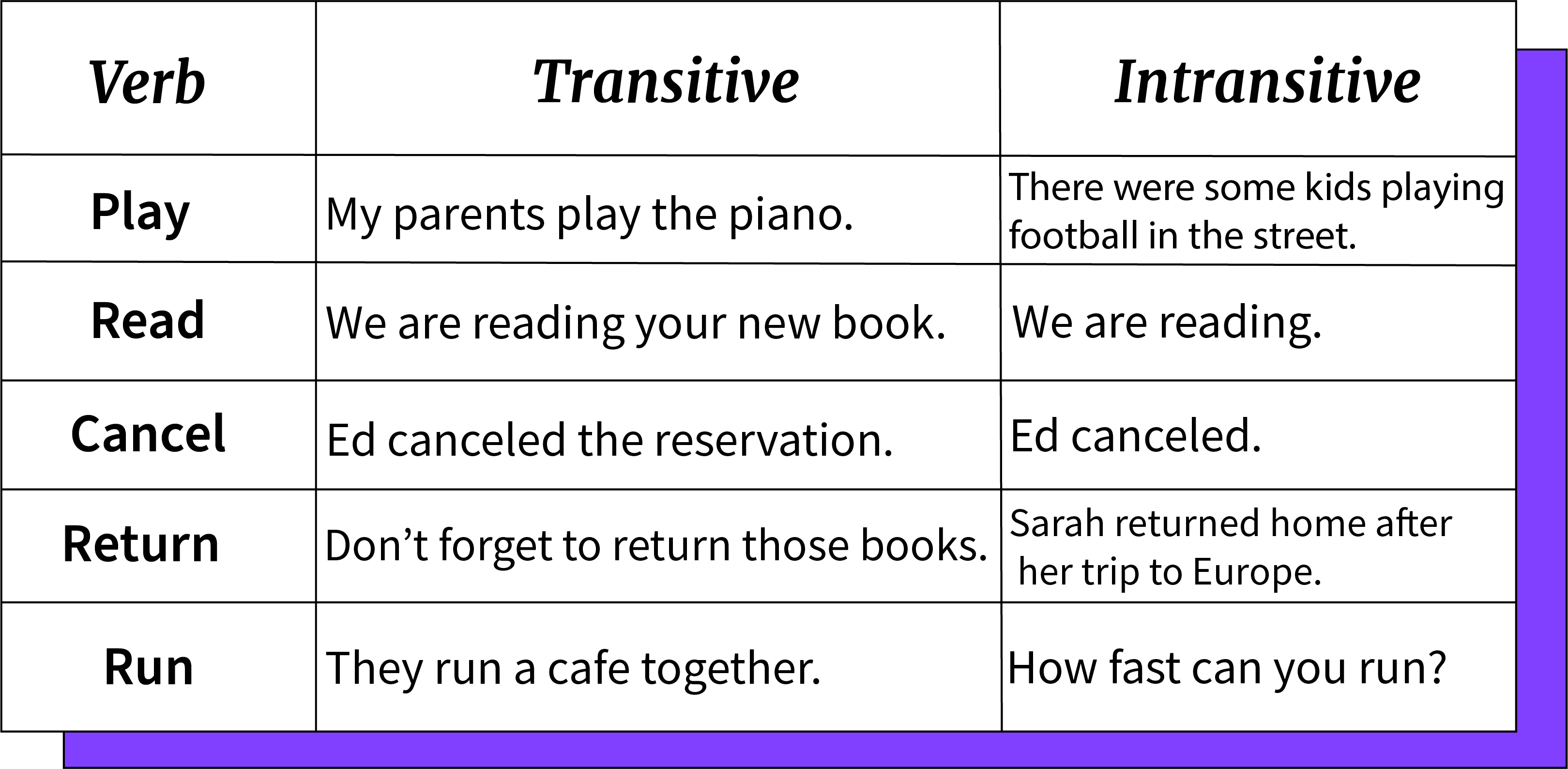
What Is the Transitive Verb? A transitive verb is the general name of verbs that can answer the question of what is used when used in a sentence. If the object to which a verb is directed is open and this object can be pointed out, this verb can be described as a transitive verb. Such verbs are verbs that we use frequently in everyday life. I am trying to understand how to calculate the transitive closure of a set and I have read several times the definition of the transitive closure but I still cannot understand some answers I see when doing questions. From my definition, the transitive closure of a set $ R^+ $ is the smallest set that is transitive and contains R.

Table of Contents

Similar and Congruent

Objects are similar to each other if they have the same shape but are different in size. We say that a six-year-old boy is similar to a 18-year-old adult man. Triangles can be similar.

Objects are congruent if they are the same shape and size. Two equilateral triangles with sides 2 meters long are congruent, since their angles and sides are all the same.

What Is The Transitive Property of Congruence?

The transitive property of congruence states that two objects that are congruent to a third object are also congruent to each other.

If giraffes have tall necks, and Melman from the movie Madagascar is a giraffe, then Melman has a long neck. This is the transitive property at work: if $a=b$ and $b=c$, then $a=c$. In geometry we can apply the transitive property to similarity and congruence.

Another way to think of it is that if one thing is like a second thing, and the second thing is like a third thing, then the first thing is like the third thing:

$A=B$

$B=C$

$\therefore A=C$

The three little dots ( $\therefore$ ), are a mathematical shorthand for 'therefore;' since $A$ is like $B$, and $B$ is like $C$, therefore$A$ is like $C$.

You use this property a lot in algebra when solving for variables. If you have two expressions with the same term in each, you can use the transitive property of congruence to connect other terms in the expressions:

$a=4+2b$

$4+2b=c$

$\therefore a=c$

Congruent Triangles Definition

In geometry, triangles can be similar and they can be congruent. Similar triangles are proportional to each other and have the same interior angles. The only difference is the length of their sides.

Proving Triangles Congruent

For two similar equilateral triangles, all interior angles will be $60°$. Say a small triangle has a side 3 meters, while a larger, similar triangle has a side 15 meters. Because the two triangles are similar, we know the sides of the larger triangle are 5 times larger than the small one.

Suppose we have two right triangles and want to see if they are similar. The sides of the small one are 3, 4, and 5 cm long. The corresponding hypotenuse of the larger triangle is 20 cm long.

Compare the ratios of the two hypotenuses:

$5:20$

Which simplifies to:

$1:4$

If the other sides have the same proportion, the two right triangles are similar. Measure and see:

$3:12$

$4:16$

All three ratios have the same proportion, $1:4$, so the two triangles are similar.

Transitive Property Of Congruent Triangles

Just as you used the transitive property of congruence to relate terms in algebraic expressions, you can also use the transitive property of congruence to connect similar triangles.

Draw a triangle, $△CAT$. Draw a triangle similar to $△CAT$ and call it $△DOG$. Now draw a triangle labeled $△ELK$ that is similar to $△DOG$.

What do you know about the relationship between $△CAT$ and $△ELK$? If $△CAT$ is similar to $△DOG$, and $△DOG$ is similar to $△ELK$, then $△CAT$ and $△ELK$ are similar to each other.

In mathematics, a special symbol is used to show similarity: ~. So we can write the entire similarity and congruence in mathematical notation:

$△CAT$ ~ $△DOG$ ~ $△ELK$

$\therefore$$△CAT$ ~ $△ELK$

Transitive Property of Congruence Examples

Knowing that for any objects, geometric or real, $Z$ ~ $A$ and $A$ ~ $P$ tells us that $Z$ ~ $P$. But how can we use that information?

Is It Transitive Calculator Word

For triangles, all the interior angles of similar triangles are congruent, because similar triangles have the same shape but different lengths of sides.

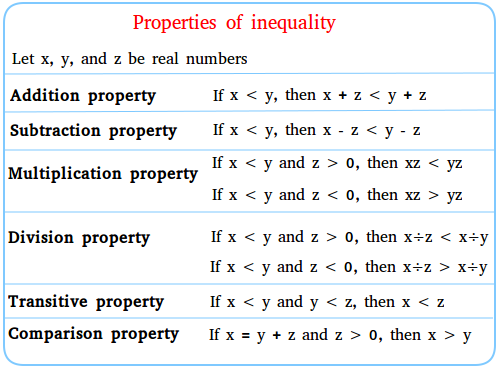
Let's make $△Z$, $△A$, and $△P$.

If $△Z$ has an angle opposite the shortest side of $37°$, $△A$also has an angle opposite its shortest side of $37°$ because we said $△Z$~ $△A$.

We also know that $△P$ has the same $37°$ in the same position because it is similar to $△A$. We also know that $△Z$~ $△P$!

Lesson Summary

By watching the video and reading the lesson, you now are able to explain the difference between congruent and similar, and define the transitive property of congruence, which states that two objects that are congruent to a third object, they are congruent to each other.

Is It Transitive Calculator Excel

You can also explain what similar triangles are, and use the transitive property to prove that size is the only difference between similar triangles.

Is It Transitive Calculator Math

Next Lesson: